
95
The second point of interest is the lower end of the
ΔK
I
range where crack growth rate
essentially decrease to zero; this is identified as the fatigue crack growth threshold,
ΔK
I, th
.
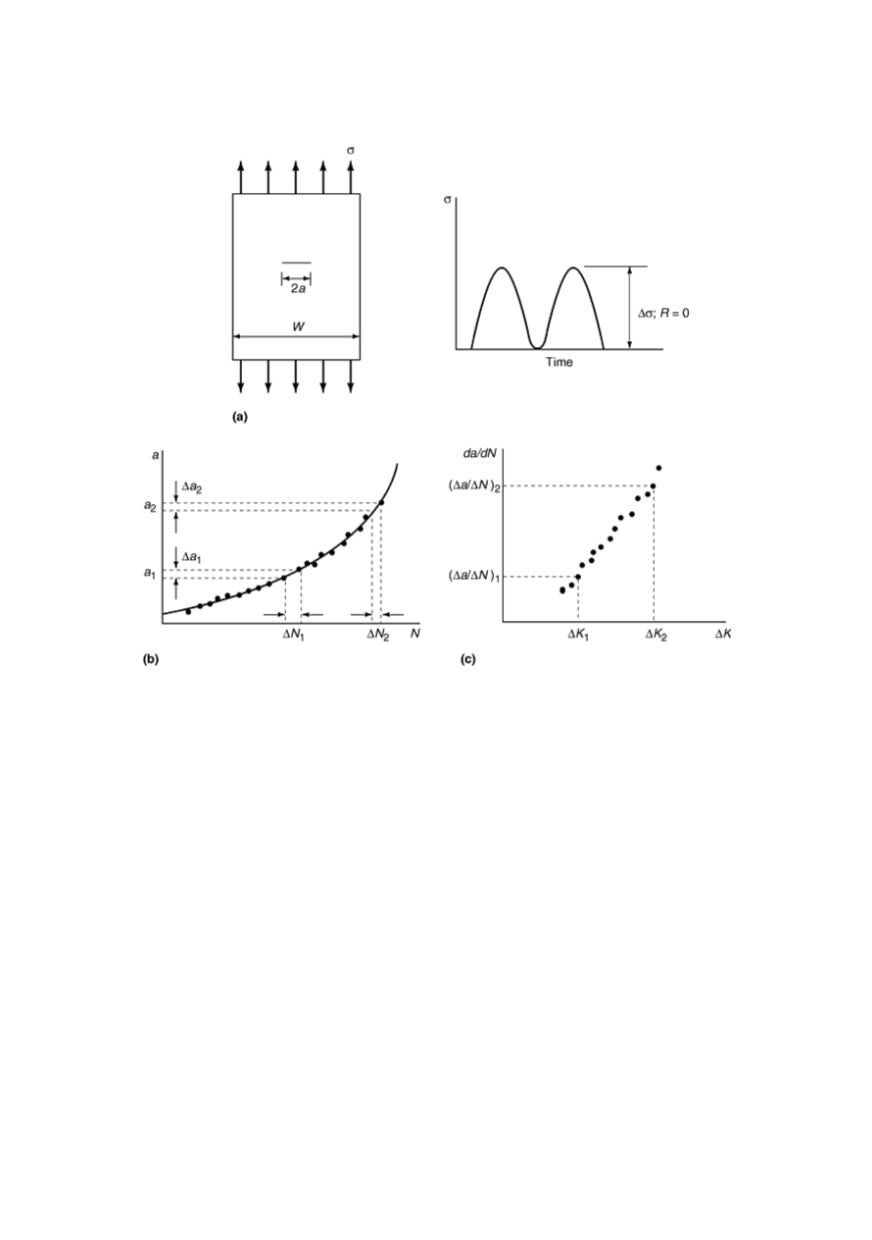
Figure 7: (a) Specimen and loading in modelling process for generating fatigue crack growth rate
(
da
/
dN
vs.
ΔK
). (b) Measured data. (c) Rate data /15/
The existence of threshold behaviour at low Δ
K
I
values is analogous, in some senses,
to the fatigue limit of some ferrous materials in
S
-
N
response. If, with the appropriate
R
ratio, the stress intensity factor range is below the threshold value, <
ΔK
I,th
, cracks will not
extend under the applied load. Thus, an assessment of nonpropagating crack can be made.
A reflection on failure criterion is appropriate here. Much as
K
I
is a quantity for asses-
sing the point of unstable fracture initiation,
ΔK
I,th
is the limit for the initiation of crack
growth (for "long" cracks) under cyclic loading. Above
ΔK
I,th
and below instability, the
criterion for subcritical extension is satisfied and the rate is as determined by the curve.
4.1. Correlation between
da
/
dN
and
ΔK
Linear elastic fracture mechanics is an analytical procedure that relates the magnitude
and distribution of stress in the vicinity of a crack tip to the remote nominal stress applied
to the structure, to the size, shape, and orientation of the crack, and to the crack growth
and fracture resistance of the material. The procedure is based on a single parameter,
K
,
called the stress-intensity factor, derived from the mathematical analysis of stress-field
equations, in the region of a crack tip. This same procedure is used to characterize fatigue
crack growth rate (
da
/
dN
) in terms of the cyclic stress-intensity factor range (
ΔK
).


















