IFMASS10

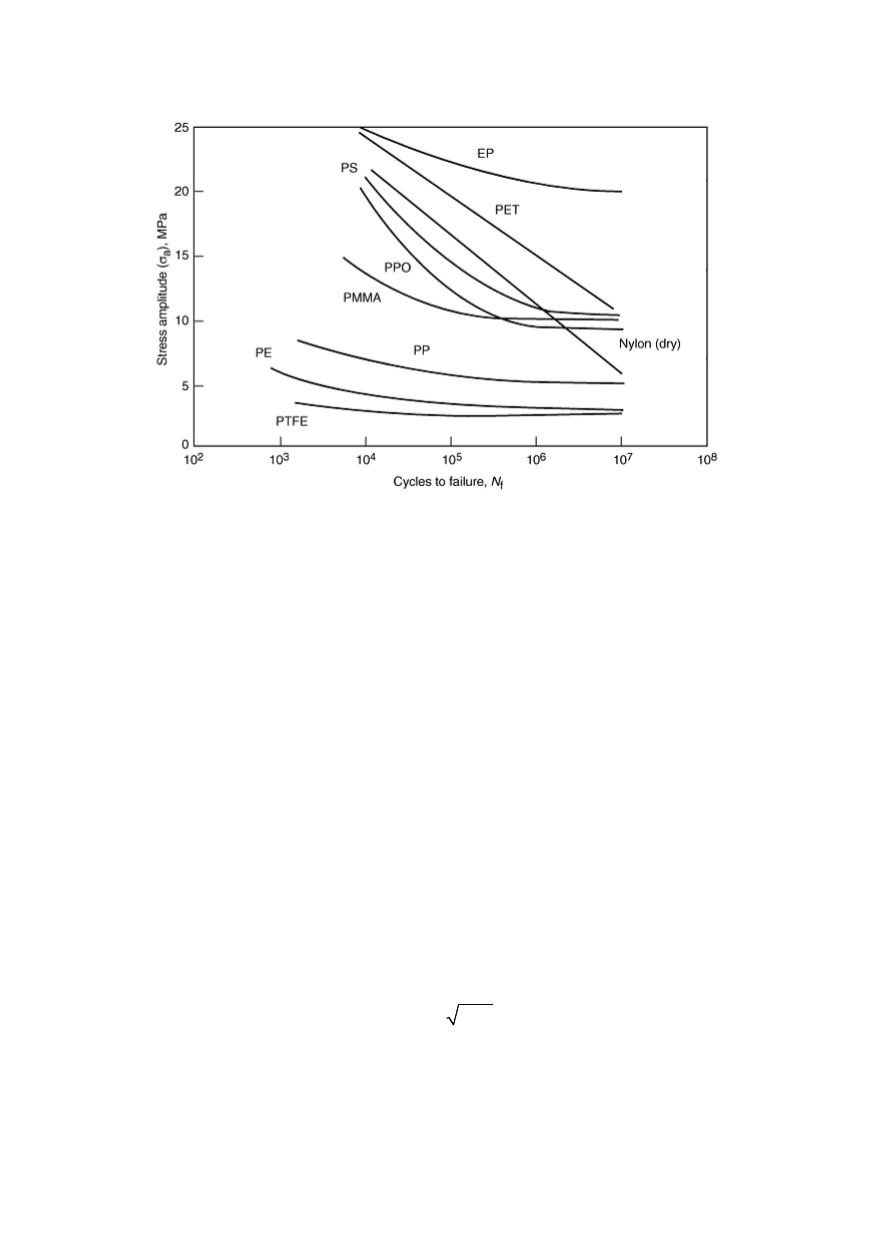
Figure 6: Typical fatigue-strength curves for different polymers (30 Hz test frequency) /14/

In multiaxial stress states the difficulty occur in identifying an appropriate "stress",

and the stress-life technique offers then a variety of advantages. Its extension using strain

as a controlling quantity is a natural progression of technology.

4. DAMAGE TOLERANT CRITERION: FRACTURE MECHANICS IN FATIGUE

The

and ε-

techniques are usually appropriate when a component or structure

can be considered a continuum (i.e. “no cracks” assumed). they can’t be applied when a

crack-like discontinuity is present. The fact that components with "cracks" may continue

to bear load is generally not acceptable using either

or ε-

methods.

One reason is the inability of the controlling quantities to allow the presence of a

crack. A brief review of basic elasticity calculations shows that both stress and strain

become infinite at a crack due to elastic stress concentration. Even induced plasticity still

leaves low tolerable loads. An alternative concept and controlling quantity is required.

That quantity is

stress intensity,

a characterization and quantification of the stress field

near the crack tip, base of linear elastic fracture mechanics (LEFM). It recognizes the

singularity of stress at the tip and provides measurable material property.

The development of fracture mechanics has roots in the early 1920s and mainly has

developed since the late 1940s and early 1950s. A very basic expression for the stress

intensity is its determination for a semi-infinite center-cracked panel having a through-

thickness crack of length 2

in a uniform stress field

that is operating normal to the

opening faces of the crack. The resulting stress intensity factor

is as follows /15/:

= ⋅

⋅

(1)

where is the far field stress responsible for opening mode loading (mode I) and

is the

crack depth in from the edge of the plate.