IFMASS10

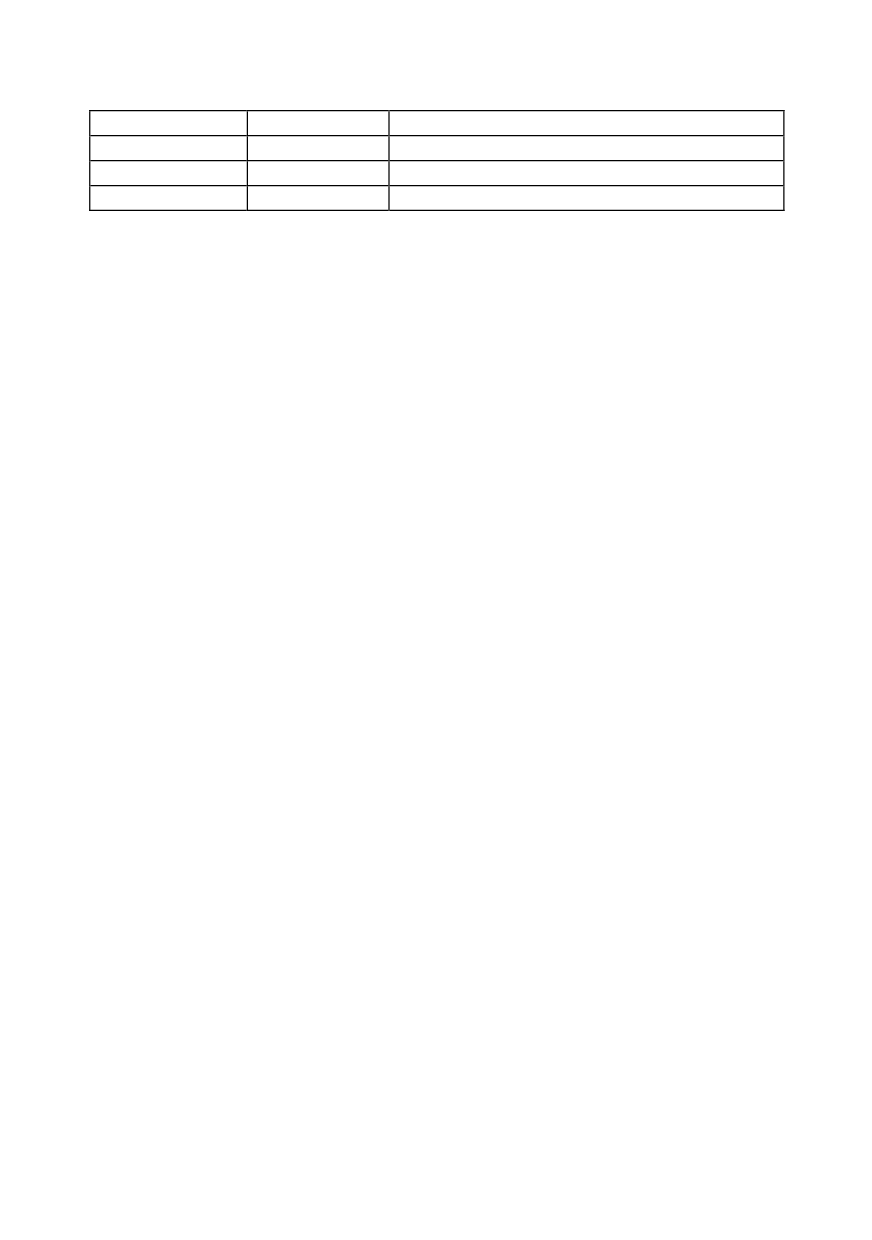
Table 1: Basic design philosophy and methodology with principal testing method

Design philosophy

Methodology

Principal testing data description

Safe-life, infinite-life

Stress-life

Stress - number of cycles,

Safe-life, finite-life

Strain-life

Strain - number of cycles,

Damage tolerant Fracture mechanics Crack growth rate-stress intensity factor range,

-Δ

The safe-life, infinite-life philosophy is the oldest approach to fatigue. It is developed

based on August Wöhler's work on railroad axles in Germany in the mid-1800s /2/. The

design method is stress-life, generally presented by

(stress vs. number of cycles to

failure). Failure in

testing is typically defined by total separation of the sample.

Applicability of the stress-life method is restricted to the homogeneous continuum,

assuming no cracks in it. However, some design guidelines for weldments, which inhe-

rently contain discontinuities, offer reduced residual life for a variety of process and joint

types that generally follow the safe-life, infinite-life approach /3/. The advantages of this

method are simplicity and ease of application. It is best applied in or near the elastic

range, addressing constant-amplitude loading situations in what has been called the long-

life (hence infinite-life) regime.

The stress-life approach is best applied to components similar to the test samples in

shape and size, important for total separation as a failure criterion. It is applicable ferrous

metals, especially steels. Other materials may not respond in a similar manner.

Through the 1940s and 1950s, mechanical designs pushed to further extremes in

advanced machinery, resulting in higher loads and stresses, including the plastic regime

of material and a more explicit consideration of finite lived components. Then the des-

cription of local events in terms of strain made more sense and resulted in the develop-

ment of assessment techniques that used strain as a determining quantity. The general

data presentation is in terms of ε-

(log strain vs. log number of cycles to failure). The

failure criterion is the detection of a "small" crack or some equivalent measure related to

a change in load-deflection response, although failure may also be defined by separation.

The use of strain is a consistent extension of the stress-life approach. As with the safe-

life, infinite-life approach, the strain-based safe-life, finite-life philosophy relies on the

"no cracks" restriction of continuous media. While more complicated, this technique

offers advantages: it includes plastic response, addresses sound approach for finite-life,

can be generalized to different geometries and variable amplitudes, and can account for a

variety of other effects. The strain-life method is better suited to handling a greater

diversity of materials. Because it does not necessarily attempt to relate to total failure

(separation) of the part, but can really initiation for final failure, it has a substantial

advantage over the stress-life method. But this method is more complex, and has more

complicated property descriptions.

The ability to generate and model both

and ε-

data effectively is very important.

From a design standpoint, there are some circumstances where inspection is not a

regularly used practice, unfeasible, or sometimes physically impossible.

In both presented techniques substantial assumption is continuity ("no cracks"). But it

is far from reality. Many components, assemblies, and structures, have crack-like discon-

tinuities induced during service or repair or as a result of primary or secondary proces-

sing, fabrication, or manufacturing, which could not be detected because of limited

sucseptibility of applied non-destructive testing equipment. However, defective parts can