
328
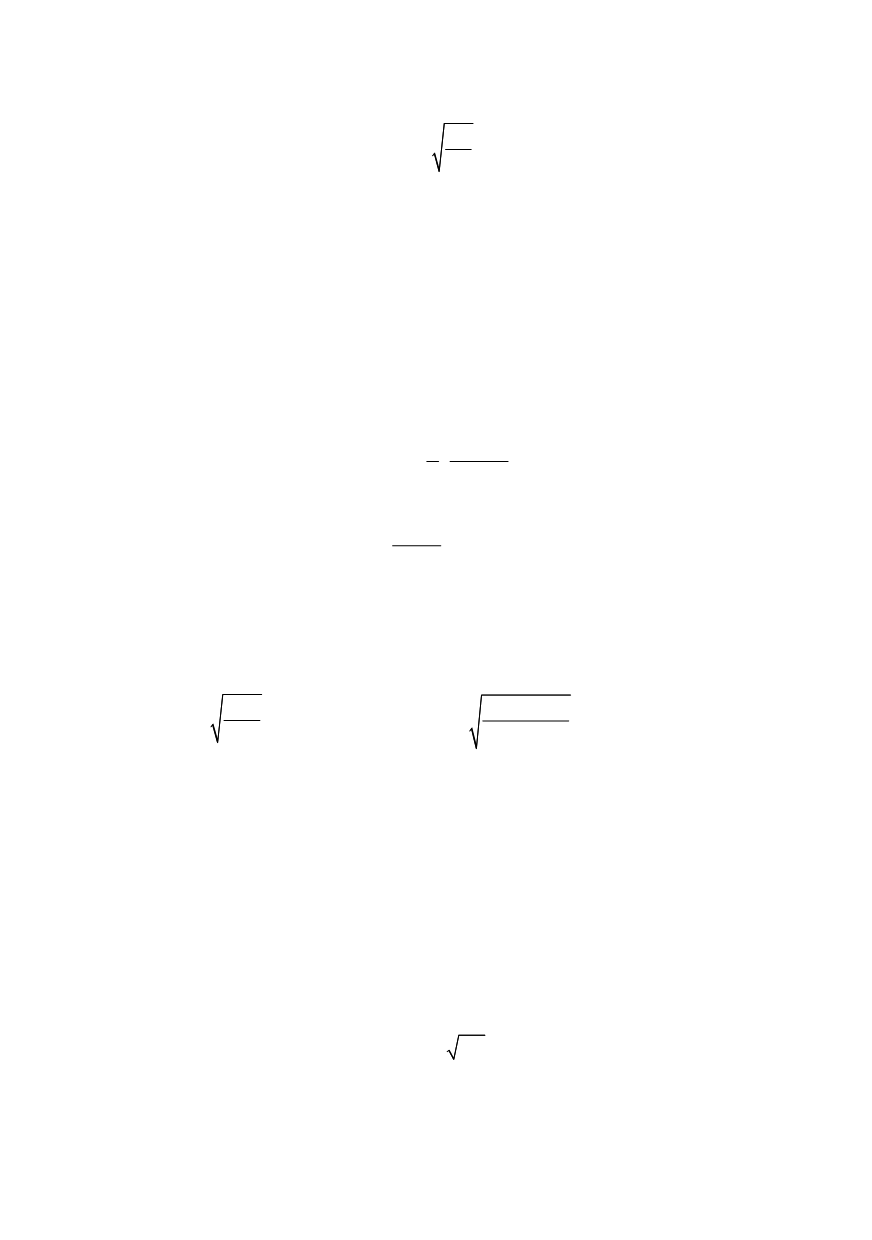
Comparing Eq. (4) and Eq. (6) following stress at fracture
σ
is obtained:
4
E
a
γ
σ
=
(7)
It can be seen immediately that, putting
γ
=
0.01
⋅
Eb
o
, as before, a defect length
2a
of
5000
b
o
, say 1
μ
m, is sufficient to lower the fracture strength by two orders of magnitude.
The Eq. (5) for the stresses around an elliptical hole was derived on the basis of linear
elasticity. The Eq. (4) is based on supposed sinusoidal atomic stress-strain curve /10/. So,
one has to have in mind that the macroscopic applied stress is correlate here to the atomic
force-displacement laws.
As it is said, these values of
∂
U/
∂
a
also represent the decrease in potential energy,
released when a crack extends by an infinitesimal amount
δ
a
under constant load.
The Griffith criterion for fracturing a body with crack of half length
a
may be visu-
alised, Fig. 8.a, drawing the way in which energy changes with crack length. The total
energy,
W
, consists of potential energy,
U
, and surface energy,
S
, and in plane stress:
2 2
1
2
2
a
W U S
a
E
σ π
γ
= + = − ⋅
+
(8)
The maximum in the total energy curve is given by a
∂
W/
∂
a=0
, i.e.
2
2
a
E
σ π
γ
=
(9)
This is depicted in Fig. 8.b, where the intersection of the line -
(
∂
U/
∂
a) =
σ
2
π
a/ E
with
that of 2
γ
is shown. The positive value of the slope
(
∂
U/
∂
a)
is defined as the potential
energy release rate for crack extension under constant load for unit thickness and signed
as
G,
presenting
crack driving force. Comparing with Eq. (3) one can derive for a given
crack length
a
the Griffith’s fracture stress:
2
F
E
a
γ
σ
π
=
(in plane stress)
2
2
1
F
E
(
)a
γ
σ
π
ν
=
−
(in plane strain)
(3a)
These expressions are similar in form to that derived for the crack tip using the solu-
tion for stress concentration /7/. Griffith’s approach ignores the details of the fracture
process at the crack tip, representing completely different procedure. Because it consi-
ders only the initial and final states and is not concerned with the details of fracturing
over the length
δ
a
, it represents only a necessary condition for fracture, which may or
may not be sufficient. Griffith’s approach could treat the change in energy involved with
the extension of a rather blunt elliptical hole and conclude that the total energy of the
system would decrease, yet the hole would not extend if there were not sufficient stress
concentrated at its end to attain the local fracture stress. The most important aspect for
next development of fracture mechanics is that the crack size,
a
, is related to applied
load, expressed through stress,
σ
, and material property, in the form of surface energy,
γ
.
The basic parameter of fracture mechanics, stress intensity factor
K
, is defined as
K Y a
σ π
=
(10)
where
Y
presents a factor of geometrical shape and final sizes of considered component.


















