
30
It can be seen from this comparison that the experiment for
J
Ic
evaluation is simpler.
Also it is clear that the requirement for
K
Ic
cannot absolutely be fulfilled, even when the
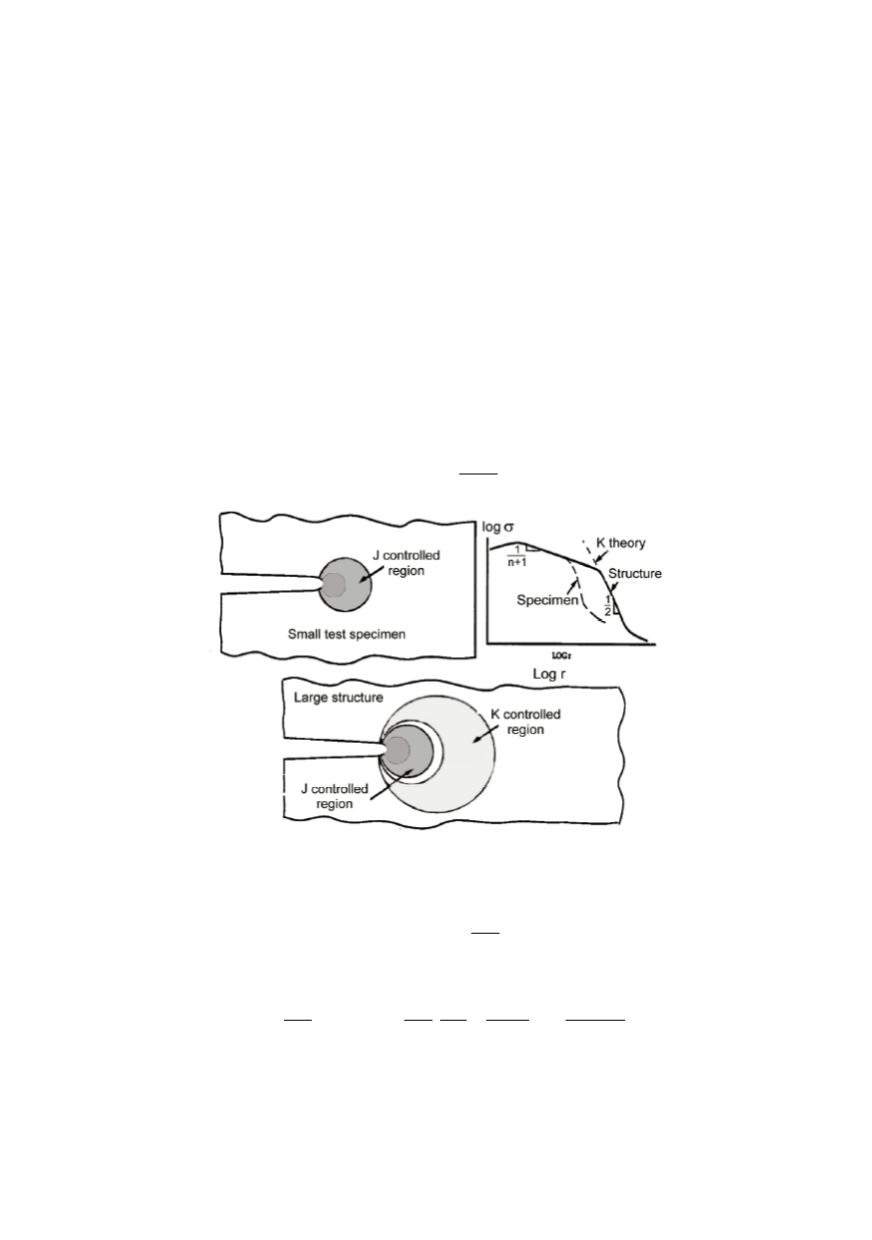
structure is in LEFM regime. This difference between test specimen and real structure is
shown in Fig. 11. Small laboratory specimen and a large structure are loaded to the same
applied
J
. The plastic zone in the small specimen is too large to be
K
controlled, but the
plastic zone for the structure is small compared to structural dimensions. Thus, the
structure has both
K
and
J
controlled regions, but the specimen has only a
J
controlled
region. Clear, in situation like this, it is possible to measure a
J
Ic
value on the small
specimen, convert this value to an equivalent
K
Ic
using Eq. (18), and apply this
K
Ic
to the
structure ignoring, however, the fact that further from the crack tip, the stress fields are
different because the stresses in the small specimen are influenced by its small size.
In spite of the situation like this, one can not expect that the parameters obtained by
the specimen of the full ligament in plastic regime can be easily transferred to the large
structure when plastic zone is limited to the relatively small area around the crack tip.
Furthermore, the name „stress intensity factor“ is in some way inadequate, because it
has dimensions, and these are, at the same time, without definite physical sense:
[ ]
3/2
N
Dim K
m
=
Figure 11: Comparison of
J
and
K
controlled areas for test specimen and large structure
Even the squaring of this relationship
2
2
3
N
Dim K
m
⎡ ⎤ = ⎣ ⎦
do not lead to the successful solution. Only after division with E-modulus (Eq. 18)
2
2 2
3
2
.
K
N m N m
Dim J G
E
Nm
m
⎡
⎤
⋅
= = =
=
⎢
⎥
⎢
⎥
⎣
⎦
=
Energy
Area
⎡
⎤
⎢
⎥
⎣
⎦
the physical sense of the notion „specific energy“
is in accordance with the theories of
fracture appearance. Previous relationship could be further cleared, if considered that one
of
K
-parameters after division by
E
gives the corresponding relative deformation
ε
(
Κ
ε
).


















