
142
and depends on number of specimen in test (Table 1). If the number of specimens is too
low the corresponding value can be even lower than the minimal value in experiments.
However, valid functional relationship is important if the safety factor is assessed in
relation to other parameters. For example, safety factor in relation to the crack size must
consider that the crack growth is exponentially dependent on crack size, so that the linear
relationship is no more typical, i.e. cannot be compared with the safety factor based on
force (load) ratio. Usual praxis of crack tolerance, where the time of next inspection is
proposed after 50 % of calculated life to the critical size, does not mean the safety factor
of 2 relative to the crack size. On the contrary, calculations and the measurements of
crack growth show that after half of life the crack growth is usually within 15-30 %.
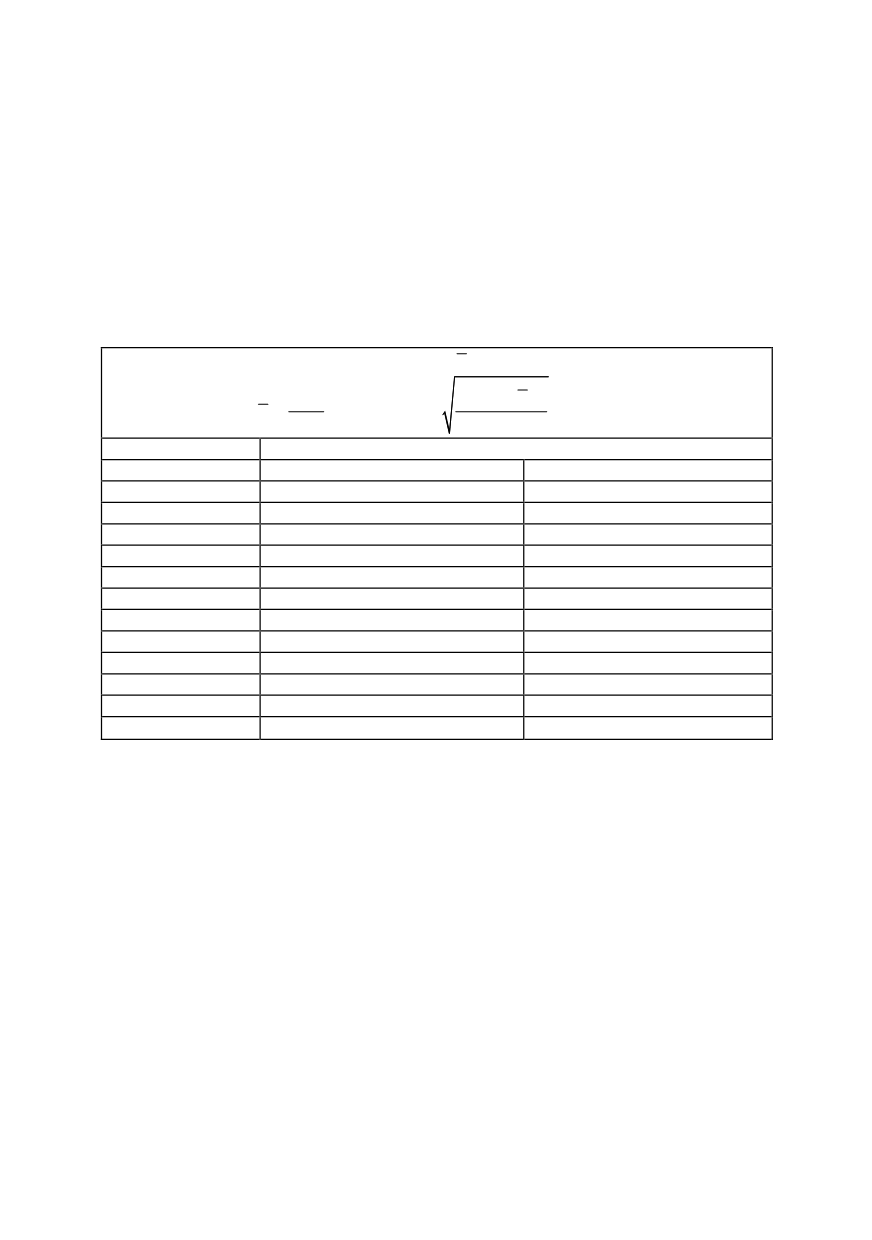
Table 1: Evaluation of minimal values for the case of normal distribution
allowable m k s
= − ⋅
where
i
x m
n
=
∑
mean value
(
)
2
1
i
x m
s
n
−
=
−
∑
and k from table
k (confidence level 95 %)
Number of tests n
99 % of probability (A values)
90 % probability (B values)
5
5.75
3.42
6
5.07
3.01
8
4.46
2.58
10
3.98
2.36
15
3.52
2.07
20
3.30
1.93
30
3.06
1.78
50
2.86
1.65
100
2.68
1.53
200
2.57
1.45
500
2.48
1.39
∞
2.33
1.28
However, safety factor based on load ratio can also be too conservative for the
structural components under strain control, and not by constant, in the range of plasticity.
In such cases is recommended to carry out “sensitivity” analysis that should show the
level of change in safety factor in dependence of influence parameters. In calculation are
all parameter varied, but so that their values stay real and, based on this the changes in
safety factor are calculated. Different codes, like BS7910, R6 and R5, SINTAP, describe
this procedure in detail. Usually is said that the safety factor has to cover all real possible
variation. Problem is that in this way can be considered only known, expected variation,
but not the unexpected one. The same problem occurs if the safety factor is assessed
based on probability and random parameters that considers random but not systematic
deviations. Independently, the factor of safety in case of fracture mechanics can never be
larger that one applied on the structure or component without crack.
Application of high safety factor brings serious problems concerning economy and
carrying capacity, as already mentioned. However, there are some situation in which
factor of safety must be higher to achieve the needed safety, like in situation of:
•
Frequent cause is uncertainty concerning material characteristics that appear due to the
insufficient number of experiments, non-homogeneity of material structure (welding


















