IFMASS10

51 / 352

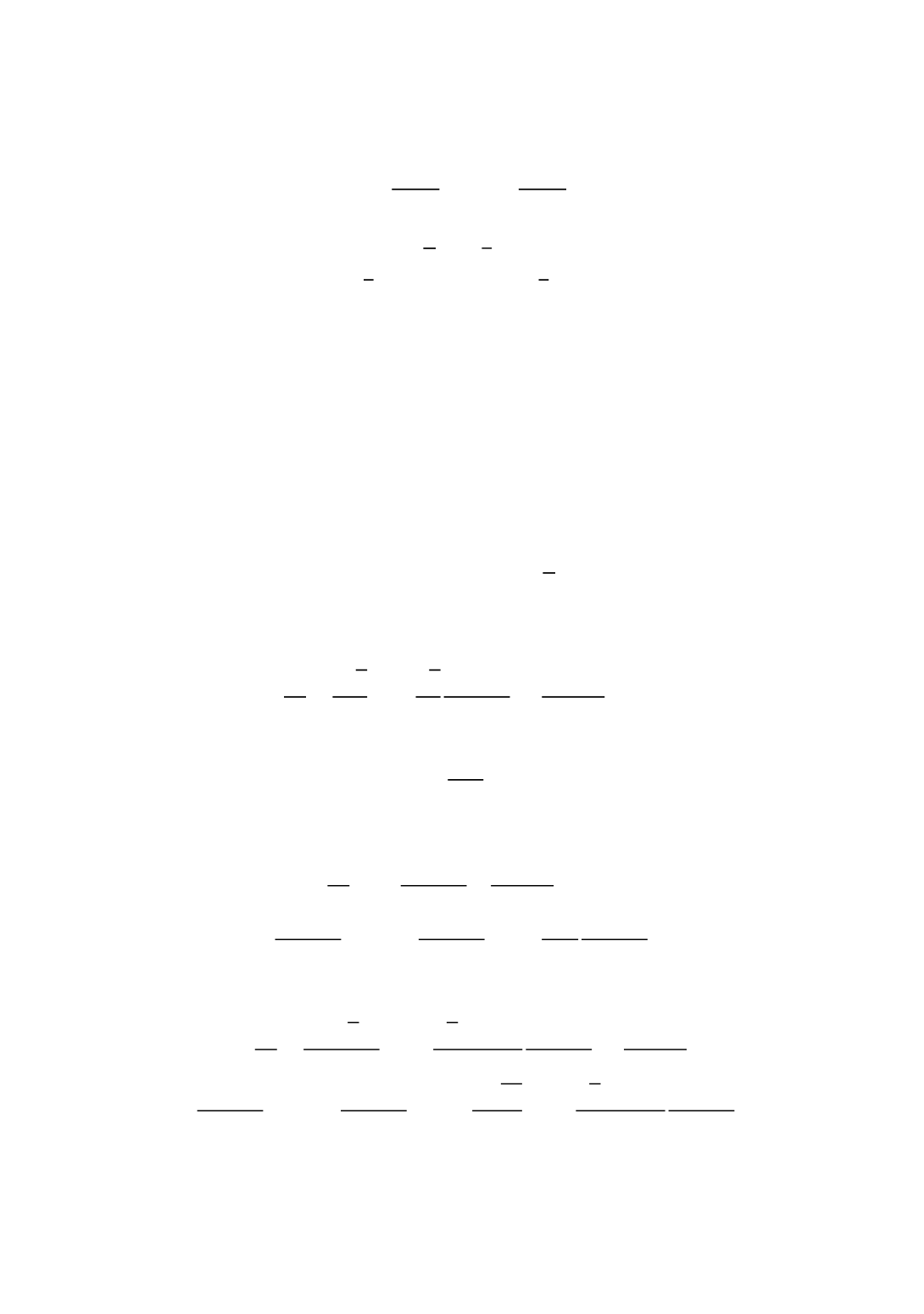
47
h௜௝௢ൌ ln ܨ௜௝ lnܨ௜௝ ௙ ൗ
can be used instead, and typical diameter of a hole and its eccentricity are denoted byR:ൌ ܽ ൅ ܾ 2 , ݁: ൌ ܽ െ ܾ ܽ ൅ ܾ .
A1-3. Strain hardening was assumed to be described by Ramberg-Osgood rule, i.e.ߪ ൌ ߪଵߝ ௡ (3)
where 1 is equivalent stress at ߝ ൌ 1 and as before ߝ ଶ ൌ 2 3⁄ ߝ ݎݐ ᇱଶ is equivalent total
strain, respectively. Moreover, Mises yield surface and associativity of flow rule was
accepted even at fracturing. As two special cases of the above hardening model he takes:
n = 0, as obvious non-hardening case, and n = 1, which he claims to be equation
corresponding to linear viscoelastic behaviour.
A1-4. Rotation of principal axes of stresses is assumed to be negligible during stress
history, which means that only principal stresses and strains considered (as mentioned at
the beginning of this subsection the analysis is not intended to describe shears but only
normal strains).
A1-5. Plastic zone covers all the considered body, and thermal effects are neglected.
Analysis
1. McClintock first derived change of his damage measure, Eq. (2), for circular cylin-
drical parallel holes in non-hardening plastic material (ߪ ൌ ܿ݋݊ ,ݐݏ i.e. n = 0).
Assuming, moreover, absence of plastic volume change for a representative circular
cylinder (Fig. 1.b) and integrating radial equilibrium equation for axisymmetric
generalized plane strain he obtained following analytical solution, /23/ - Eq. (11):ln ܴ ܴ଴ ൌ ߝҧ√3 2sinh ቈ √3 2 ߪ௔ ൅ ߪ௕ ߪത ቉ ൅ ߝ௔ ൅ ߝ௕ 2 (4)
2. On the other hand, for a linear viscoelastic material obeying the evolution equationߝሶᇱ ൌ 1 2#ߤ T' (5)
where #= const is viscosity coefficient, under the condition of constant stress deviator
we haveߪഥ ൌ ߪതݐ 3# ⁄ . The corresponding solutions, according to /17/, now readln ܴ ܴ଴ ൌ 3ߝ ҧ ߪ௔ ൅ ߪ௕ ߪത ൅ߝ௔ ൅ ߝ௕ 2 (6)
݁ ൌ ߪ௔ െ ߪ௕ ߪ௔ ൅ ߪ௕ ൅ ൬݁଴ െ ߪ௔ െ ߪ௕ ߪ௔ ൅ ߪ௕ ൰ ݁ݔ ݌ ൤ െ3ߝ ҧ 2ߪ௔ ൅ ߪ௕ ߪത ൨ (7)
3. Taking Eqs. (1) and (5) as two limiting cases of the power hardening Eq. (3),
corresponding to n =0 and n =1, respectively, next interpolation is introduced in /23/
ln ܴ ܴ଴ ൌ ߳ҧ√3 2ሺ1 െ ݊ሻ ݏ݄݅݊ ቈ √3ሺ1 െ ݊ሻ 2 ߪ௔ ൅ ߪ௕ ߪത ቉ ൅ ߝ௔ ൅ ߝ௕ 2 (8)
݁ ൌ ߪ௔ െ ߪ௕ ߪ௔ ൅ ߪ௕ ൅ ൬݁଴ െ ߪ௔ െ ߪ௕ ߪ௔ ൅ ߪ௕ ൰ ݁ݔ݌ ቆ െ√3ߝҧ 1 െ ݊ݏ݄݅݊ ቈ √3ሺ1 െ ݊ሻ 2 ߪ௔ ൅ ߪ௕ ߪത ቉ቇ (9)
Which, reduces to Eqs. (4), (6) - (7) in the mentioned limiting cases. By means of
identity ܾ ൌ ܴሺ1 െ ݁ሻ and of logarithmic (true) strain ߝ ௕ ൌ lnሺ݈௕ ݈௕଴ ⁄ ሻ from Eq. (2)