
231
(iii) Elements with potential energy greater than the kinetic energy. In this case
stiffness should be increased, by increasing moment of inertia of corresponding section.
Proper example here is fixed element. This change can produce appropriate eigenvalue.
(iv) Elements with kinetic and potential energy, which values are not negligible
comparing to other elements. These elements are the most difficult to be reanalysed, but
they are the most frequent. A simple supported beam between supports is typical
example. Way of modification depends of the local situation. Based on analysed
examples, one type of modification would be increasing stiffness on these elements
without increasing mass, or just reducing mass, if possible. It is possible to achieve by
involving elements with larger cross section moment of inertia without changing mass or
involving elements with the same moment of inertia but allying another material. An
alternative is to use new elements assuring increased stiffness, and with increased
displacements the kinetic energy will be also increased. The basic aim of this modifica-
tion is to enhance eigenvalues. If the energy increment is reduced, this procedure
exhibited the convergence, as required as a proof for successful procedure.
4. EXAMPLES OF PERFORMED REANALYSIS
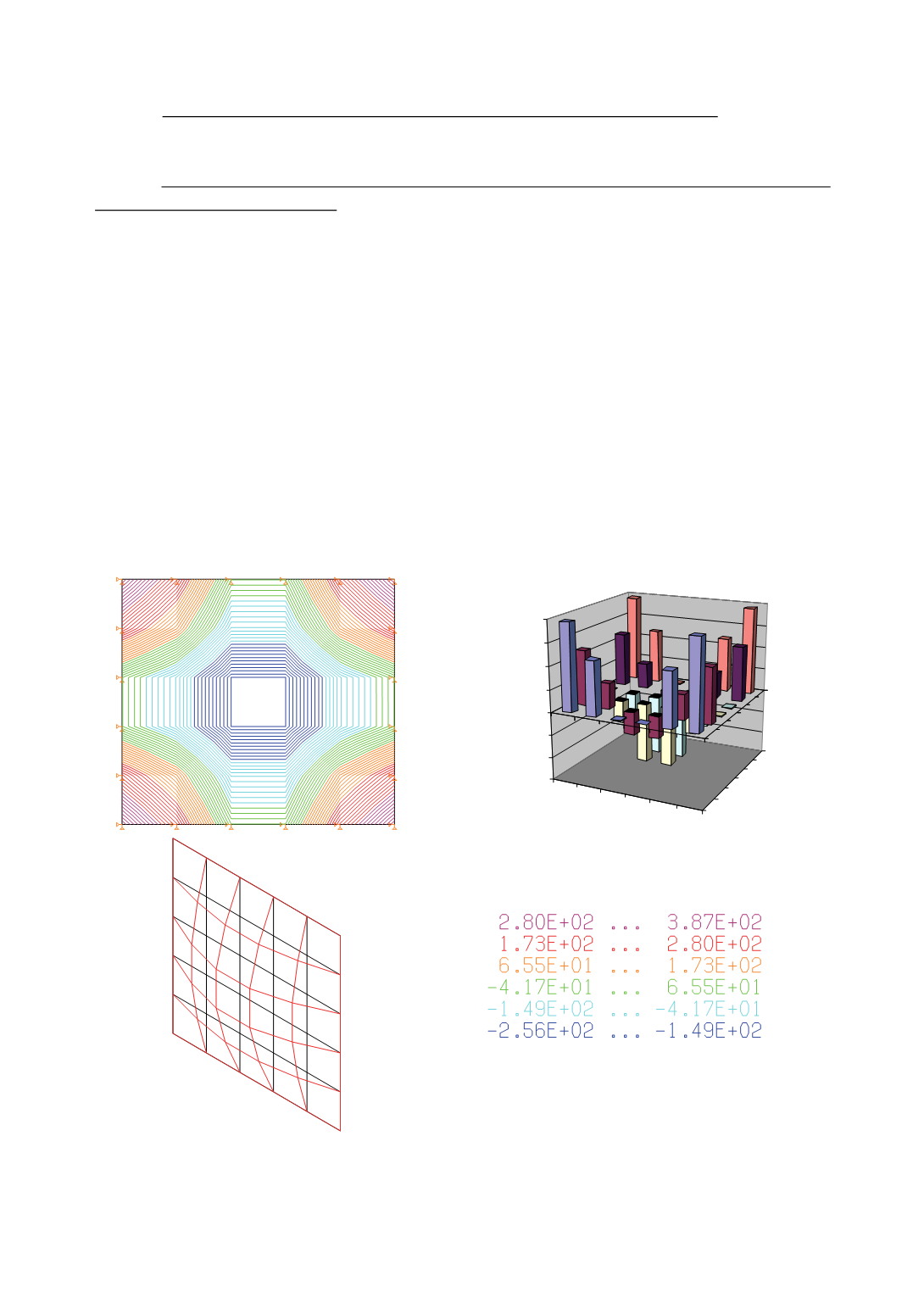
In this analysis thin plates have been considered. The plate, 100 cm x 100 cm in size,
and 1 cm thick is considered as initial plate. It was supported by hinges on each side,
(Fig. 3), or it was fixed along the sides (Fig. 4).
1 2 3 4 5 6
S1
S4
-3.00E+02
-2.00E+02
-1.00E+02
0.00E+00
1.00E+02
2.00E+02
3.00E+02
4.00E+02
Figure 3: The square plate supported by hinges along the each side. Distribution of potential and
kinetic energy increments, kNxcm, for initial and arbitrary modified plate is presented. First
frequency of the initial plate is
f
01
= 48.63 Hz, and for arbitrary modified plate
f
01
’
= 53.5 Hz.


















