
226
freedom or change of material. Several procedures for the dynamic reanalysis of
structures concern the changes encountered by the dynamic properties of the structures
when a modification is involved. Usually, the dynamic properties under consideration are
the modal parameters, and for that several studies have been addressed to the modal
reanalysis of linear structures. The perturbation approach has been utilized by Inamura /1/
and by Ki /2/ for the evaluation of the approximate eigen properties of the modified
structural system. Rayleigh’s method has been utilized by Wang and Pilkey /3/ and
Hodges /4/ for the evaluation of the approximate eigenvalues and eigenvectors of the
modified structure, taking into account the results of the initial modal analysis of the
original structure. Nair, Keane and Langley /5/ proposed a method to improve first-order
approximation of eigenvalues and eigenvectors. The reanalysis problems attract the
attention recently, and many papers are devoted to this topic /6-28/.
The main goal of dynamic reanalysis is to provide numerical procedures to evaluate
the structural response after modifications of design variables. The general problem of
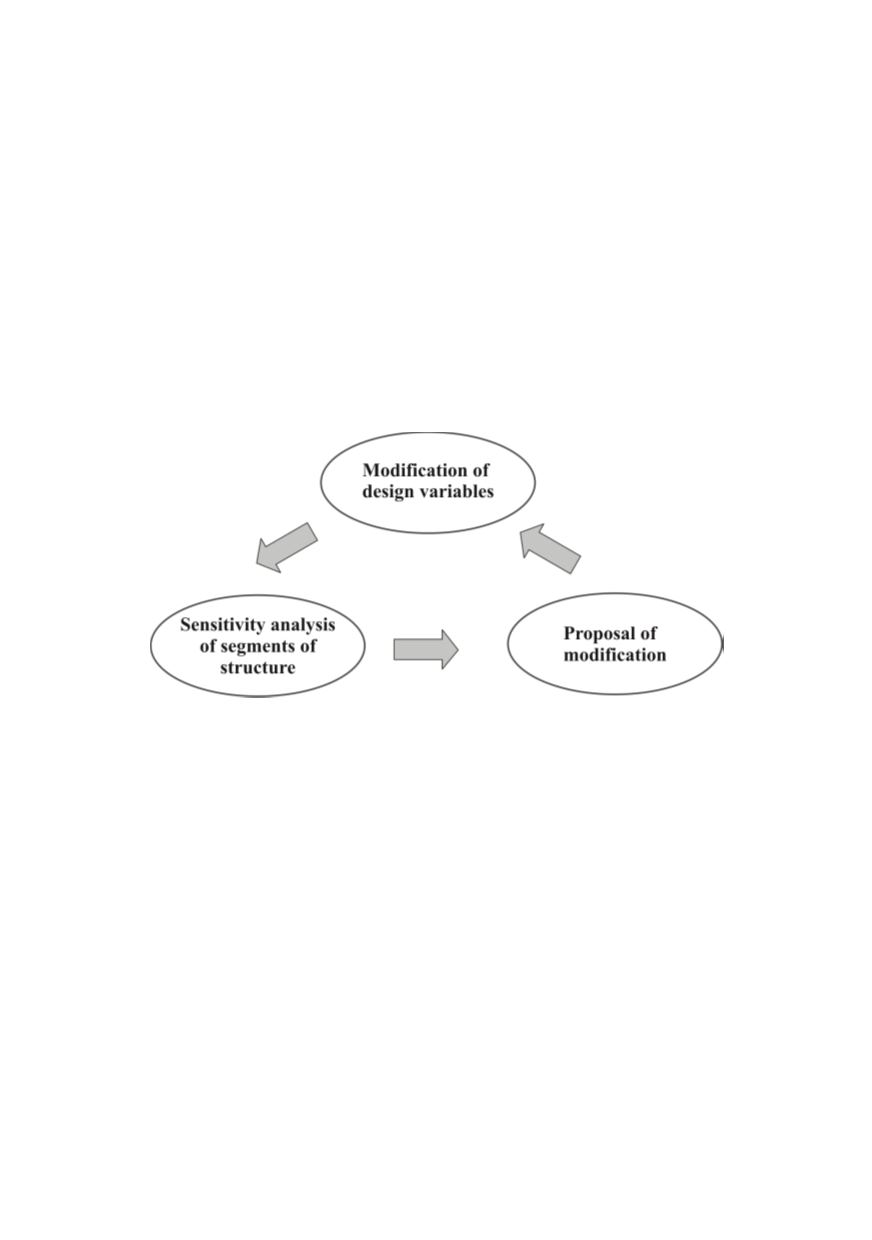
dynamic reanalysis is presented in Fig. 2 and consists of three important aspects.
Figure 2: Triangle of dynamic reanalysis
The aim of this presentation is to show how the change of support conditions can
improve dynamic characteristics of a structure. Structural model is the most important
prerequisite for this analysis, because the distribution of potential and kinetic energy in
every finite element (FE) of model is necessary for the evaluation of reanalysis success.
Appropriate finite element or analytical model of mechanical structure is important for
the successful structural integrity analysis. In practice, a high degree of confidence can be
achieved with such an analytical FE model when its dynamic response is confirmed by an
experimental analysis. However, updating the FE model or identifying the analytical
model directly is usually not the main objective of structural vibration analysis because
there are many situations when the dynamic response of the mechanical structure does not
satisfy the requirement specified by the structural analyst (designer). In such situations,
the dynamic response of the mechanical structure has to be altered either by controlling
the input data to the structure, or by changing the dynamic characteristics of the structure.
The accepted inputs often result from interaction between the structure and environment
and for that can’t be easily controlled. When this is the case, the ability to alter the
structural response by redesigning the dynamic characteristics of the structure is of prime
significance. The use of structural reanalysis techniques to obtain the optimum condition
of FE model of a mechanical structure has been extended considerably in recent years.


















